Efectos de la presión hidrostática sobre los modos defectivos en un cristal fotónico bidimensional semiconductor con geometría hexagonal

Resumen

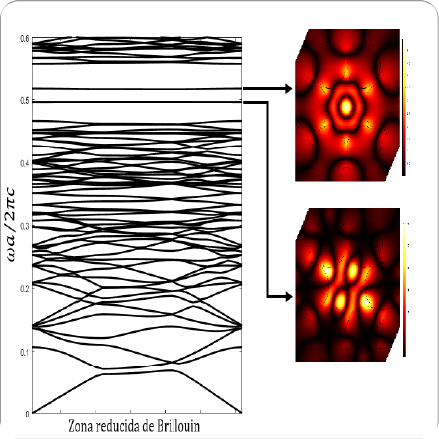
Las propiedades ópticas de las estructuras caracterizadas mediante una función dieléctrica periódica como los cristales fotónicos (CFs), ha despertado interés por sus aplicaciones en diversos campos de la óptica y la optoelectrónica. Cuando la periodicidad de los CFs se rompe por la inserción de defectos, permite el confinamiento de modos de luz. En esta contribución, utilizando el método de expansión en ondas planas para el modo de polarización transversal magnética y el Software computacional MATLAB, se estudia el efecto de la presión hidrostática sobre la estructura de bandas en un cristal fotónico bidimensional (CF-2D) con red hexagonal, compuesto por agujeros de aire en un fondo de material semiconductor de
fosfuro de galio (GaP), que se caracteriza por tener el índice de refracción más alto entre los semiconductores binarios y su rango de transmisión se utiliza en aplicaciones de luz visible e infrarrojo cercano. Inicialmente, se estudia la relación de dispersión del cristal fotónico regular (sin defecto) y se analiza la zona de Banda Fotónica Prohibida (BFP); posteriormente se
aumenta la presión hidrostática, generando que la estructura de banda fotónica presente un desplazamiento a regiones de alta frecuencia. Posteriormente, utilizando la técnica de la supercelda se considera un defecto puntual dentro de la estructura, que reemplaza un agujero de aire por material semiconductor, que genera estados permitidos o modos de defecto en el interior del bandgap fotónico. Al aumentar la presión hidrostática se observa que el ancho del bandgap fotónico permanece inalterado y los modos de defecto presentan un desplazamiento a valores de altas frecuencias.