Aplicación de una función límite de distribución hipergeométrica negativa en la valoración de opciones
DOI:
https://doi.org/10.24054/bistua.v20i2.1497Palabras clave:
Negative hyper geometric distribution, wealth equation and optionResumen
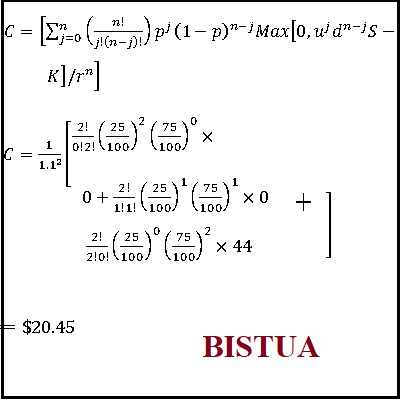
Este trabajo presenta la función límite de una distribución hipergeométrica negativa que se aplica en la valoración de opciones utilizando la ecuación de riqueza y algunas herramientas de martingala. Este artículo presenta un modelo de tiempo discreto simple en comparación con otro modelo existente. Este trabajo concluye que el límite de la hipergeometría negativa se puede asociar con términos financieros que se pueden usar para evaluar los valores de las opciones (sin dividendos), lo que da el mismo valor numérico que el modelo CRR.
Descargas
Referencias
A. Mehra, S.D Chandra and R. Khemchandari, Financial Mathematics An introduction, Narosa Publishing House, India first edition pp 49-75, 2013.
F.I Cheng and C.I. Alice ,Application of Binomial distribution to evaluate call option (finance) handbook of quantitative finance and risk management availabl e onnline.www.springer.com 2010
J.C Cox, S.A Ross. and M. Rubinstein ,Option pricing: A Simplified Approach journal of financial Economic 7(3):2-34 ,1979.
R. Stockbridge,The discrete Binomial model for option pricing ,Phdprogram in Applied mathematics, available online www.sematicscholar.org. 2008.
K..Teerapabolarn An improved Binomial distribution to approximate the negative hypergeometric distribution, International Journal of pure and applied mathematics 91(1):77-81. 2014
M. B. Zeina, A. Hatip, Neutrosophic random variables, Neutrosophic Sets and Systems, 39, 44-52, 2021.
C. Granados,New results on neutrosophic random variables Neutrosophic Sets and Systems, 47, 286-297, 2021.
C. Granados, J. Sanabria, On independence neutrosophic random variables, Neutrosophic Sets and Systems, 47, 541-557, 2021.
C. Olunkwa, B.O. Osu, C. Granados, Mean variance portfolio selection problem with multiscale stochastic volatility, Prospectiva 20(2)(2022), 1-11
Archivos adicionales
Publicado
Número
Sección
Licencia
Derechos de autor 2022 © Autores; Licencia Universidad de Pamplona.

Esta obra está bajo una licencia internacional Creative Commons Atribución-NoComercial-SinDerivadas 4.0.
© Autores; Licencia Universidad de Pamplona.





