Bessel polynomials by context-free grammars
Polinomios de Bessel mediante gramáticas independientes del contexto
DOI:
https://doi.org/10.24054/bistua.v22i2.2932Keywords:
Bessel polynomials, formal derivative operator, context-free grammarsAbstract
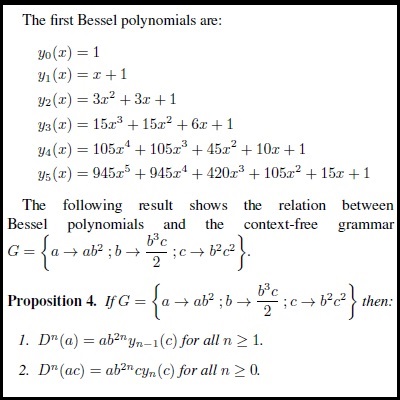
The Bessel polynomials are an orthogonal family of polynomials yn(x) introduced as solutions of the second-order differential equation x2y''+2(x+1)y'=n(n+1)y, they satisfy the recurrence relation yn(x)=(2n-1)xyn-1(x)+yn-2(x), where y1(x)=x+1 y y0(x)=1. In terms of derivatives, Bessel polynomials can be described by the recurrence yn(x)=(nx+1)yn-1(x) + x2y'n-1(x). In this paper, we study the connection between Bessel polynomials and context-free grammars through the formal derivative operator, and we prove some identities of this family of polynomials.
Downloads
References
Chen, W.Y., Context-free grammars, differential operators and formal power series. Theoretical Computer Science 117 (1993) 113-129. https://doi.org/10.1016/0304-3975(93)90307-F
Chen W.Y., Fu A.M., Context-free grammars for permutations and increasing trees. Advances in Applied Mathematics 82 (2017) 58-82. https://doi.org/10.1016/j.aam.2016.07.003
Chen W.Y., Yang H.R., A context-free grammar for the Ramanujan-Shor polynomials. Advances in Applied Mathematics 126 (2021) 101908. https://doi.org/10.1016/j.aam.2019.04.005
Doha E.H., Ahmed H.M., On the coefficients of integrated expansions of Bessel polynomials. Journal of Computational and Applied Mathematics 187(1) (2006), 58-71. https://doi.org/10.1016/j.cam.2005.03.036
Gould H., Quaintance J., Double fun with double factorials. Mathematics Magazine 85(3) (2012) 177-192. https://doi.org/10.4169/math.mag.85.3.177
Hao R.X., Wang L.X., Yang H.R., Context-free grammars for triangular arrays. Acta Mathematica Sinica 31 (2015) 445-455. https://doi.org/10.1007/s10114-015-4209-5
Krall H.L., Frink O., A new class of orthogonal polynomials: The Bessel polynomials. Transactions of the American Mathematical Society 65(1) (1949) 100-115.
Ma S.M., Some combinatorial arrays generated by context-free grammars. European journal of combinatorics 34(7) (2013) 1081-1091. https://doi.org/10.1016/j.ejc.2013.03.002
Ma S.M., Ma J., Yeh Y.N. Zhu B.X., Context-free grammars for several polynomials associated with Eulerian polynomials. The Electronic Journal of Combinatorics 25(1) (2018) P1.31. https://doi.org/10.37236/6979
Ma S.M., Yeh Y.N., Eulerian polynomials, Stirling permutations of the second kind and perfect matchings. The Electronic Journal of Combinatorics 24(4) (2017) P4.27. https://doi.org/10.37236/7288
Ma S.M., Mansour T., Schork M., Normal ordering problem and the extensions of the Stirling grammar. Russian Journal of Mathematical Physics 21 (2014) 242-255. https://doi.org/10.1134/S1061920814020095
Rather N.A., Wani M.S., Dar I., On the Enestr"om-Kakeya theorem. Applied Mathematics E-Notes 22 (2022), 660-667.
Triana J., De Castro R., Grammars and multifactorial numbers. Global Journal of Pure and Applied Mathematics 15(3) (2019) 251-259.
Triana J., De Castro R., The formal derivative operator and multifactorial numbers. Revista Colombiana de Matem'aticas 53(2) (2019) 125-137. https://doi.org/10.15446/recolma.v53n2.85522
Triana J., $r$-Stirling numbers of the second kind through context-free grammars. Journal of automata languages and combinatorics 27(4) (2022) 323-333. https://doi.org/10.25596/jalc-2022-323
Zhou R.R., Yeh, J., Ren F., Context-free grammars for several triangular arrays. Axioms 11(6) (2022) 1-10. https://doi.org/10.3390/axioms11060297
Zhu B.X., Yeh Y.N., Lu Q., Context-free grammars, generating functions and combinatorial arrays. European journal of combinatorics 78 (2019) 236-255. https://doi.org/10.1016/j.ejc.2019.02.007
Additional Files
Published
Versions
- 2024-10-24 (2)
- 2024-08-21 (1)
Issue
Section
License
Copyright (c) 2024 © Autores; Licencia Universidad de Pamplona

This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.
© Autores; Licencia Universidad de Pamplona





